The Equation for a Cubic Spline
- We will create a succession of cubic splines over successive intervals of the data (See Fig. 4).
- Each spline must join with its neighbouring cubic polynomials at the knots where they join with the same slope and curvature.
- We write the equation for a cubic polynomial,
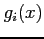 , in the
, in the 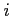 th interval, between points
th interval, between points
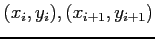 .
.
- It looks like the solid curve shown here.
- The dashed curves are other cubic spline polynomials. It has this equation:
- Thus, the cubic spline function we want is of the form
- and meets these conditions:
- Equations say that the cubic spline fits to each of the points Eq. 1, is continuous Eq. 2, and is continuous in slope and curvature Eq. 3 and Eq. 4, throughout the region spanned by the points.
Cem Ozdogan
2010-12-06